Linear models with a single predictor

Lecture 15

Dr. Mine Çetinkaya-Rundel

Duke University
STA 199 - Fall 2024

October 24, 2024

Warm-up

While you wait…

Go to your ae project in RStudio.
Make sure all of your changes up to this point are committed and pushed, i.e., there’s nothing left in your Git pane.
Click Pull to get today’s application exercise file: ae-13-modeling-penguins.qmd.
Wait till the you’re prompted to work on the application exercise during class before editing the file.

Announcements

AE 12 (Tuesday’s AE) solutions posted for extra practice, AE 13 (today’s AE) incorporates bits of AE 12 we didn’t get to on Tuesday
Academic misconduct issues so far:
- Uncited use of outside materials, including (and especially) AI tools
- Same work turned in by multiple students

Correlation vs. causation

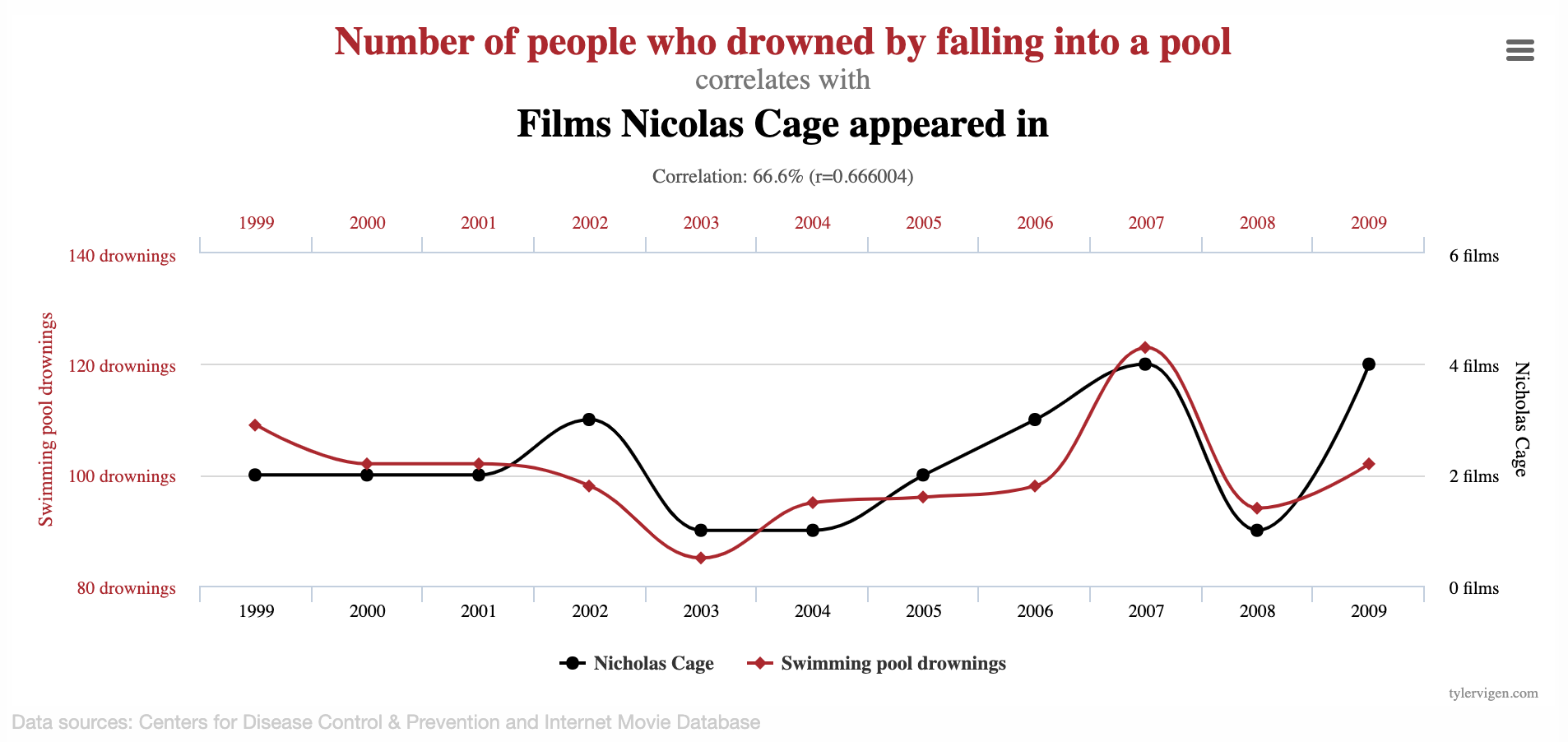
Spurious correlations

Spurious correlations

Linear regression with a single predictor

Data prep

Rename Rotten Tomatoes columns as critics and audience
Rename the dataset as movie_scores

movie_scores <- fandango |>
  rename(
    critics = rottentomatoes, 
    audience = rottentomatoes_user
  )

Data overview

movie_scores |>
  select(critics, audience)

# A tibble: 146 × 2
   critics audience
     <int>    <int>
 1      74       86
 2      85       80
 3      80       90
 4      18       84
 5      14       28
 6      63       62
 7      42       53
 8      86       64
 9      99       82
10      89       87
# ℹ 136 more rows

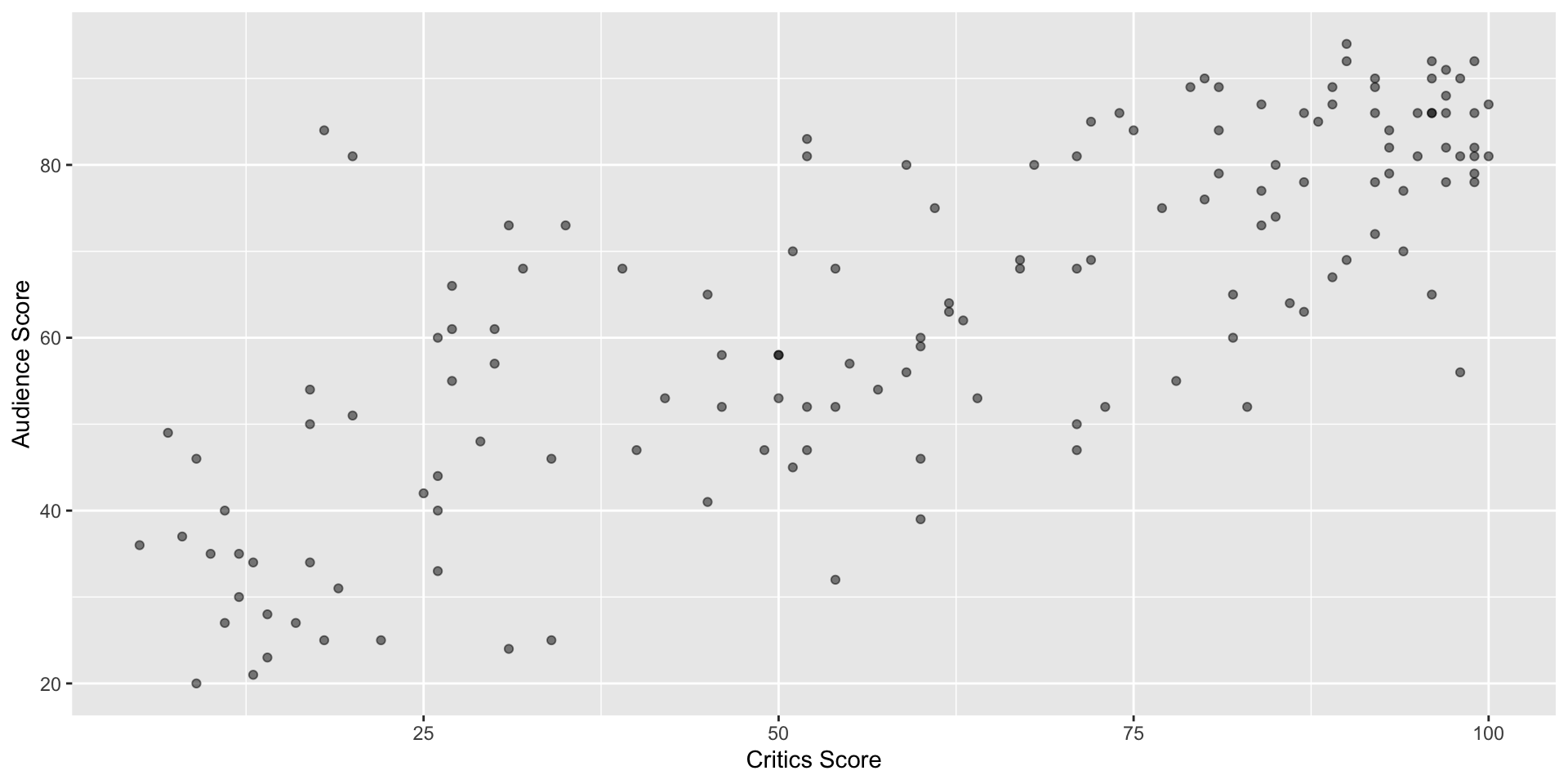
Data visualization

Regression model

A regression model is a function that describes the relationship between the outcome, \(Y\), and the predictor, \(X\).

\[\begin{aligned} Y &= \color{black}{\textbf{Model}} + \text{Error} \\[8pt] &= \color{black}{\mathbf{f(X)}} + \epsilon \\[8pt] &= \color{black}{\boldsymbol{\mu_{Y|X}}} + \epsilon \end{aligned}\]

Regression model

\[ \begin{aligned} Y &= \color{#325b74}{\textbf{Model}} + \text{Error} \\[8pt] &= \color{#325b74}{\mathbf{f(X)}} + \epsilon \\[8pt] &= \color{#325b74}{\boldsymbol{\mu_{Y|X}}} + \epsilon \end{aligned} \]

Simple linear regression

Use simple linear regression to model the relationship between a quantitative outcome (\(Y\)) and a single quantitative predictor (\(X\)): \[\Large{Y = \beta_0 + \beta_1 X + \epsilon}\]

\(\beta_1\): True slope of the relationship between \(X\) and \(Y\)
\(\beta_0\): True intercept of the relationship between \(X\) and \(Y\)
\(\epsilon\): Error (residual)

Simple linear regression

\[\Large{\hat{Y} = b_0 + b_1 X}\]

\(b_1\): Estimated slope of the relationship between \(X\) and \(Y\)
\(b_0\): Estimated intercept of the relationship between \(X\) and \(Y\)
No error term!

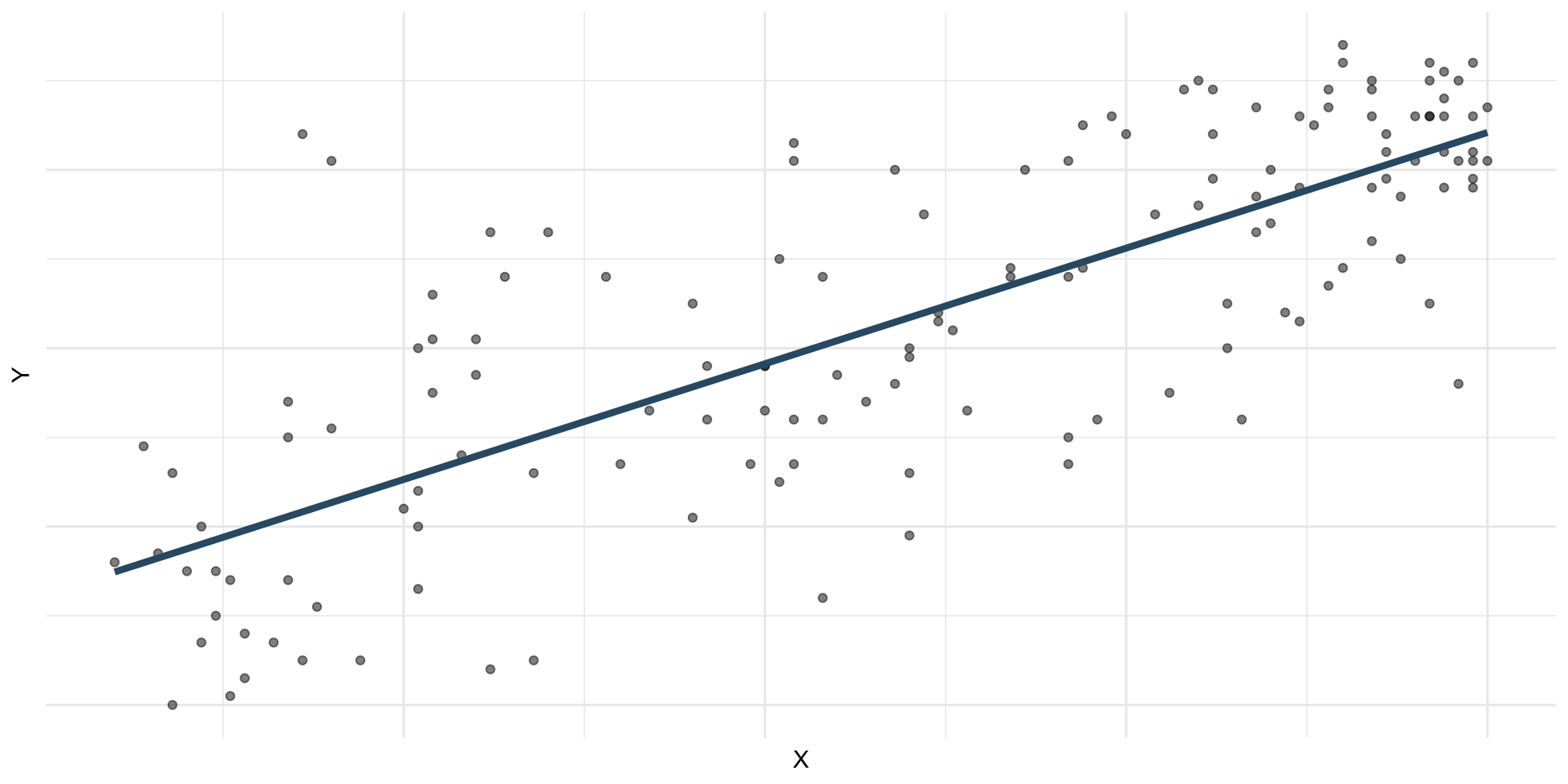
Choosing values for \(b_1\) and \(b_0\)

Residuals

\[\text{residual} = \text{observed} - \text{predicted} = y - \hat{y}\]

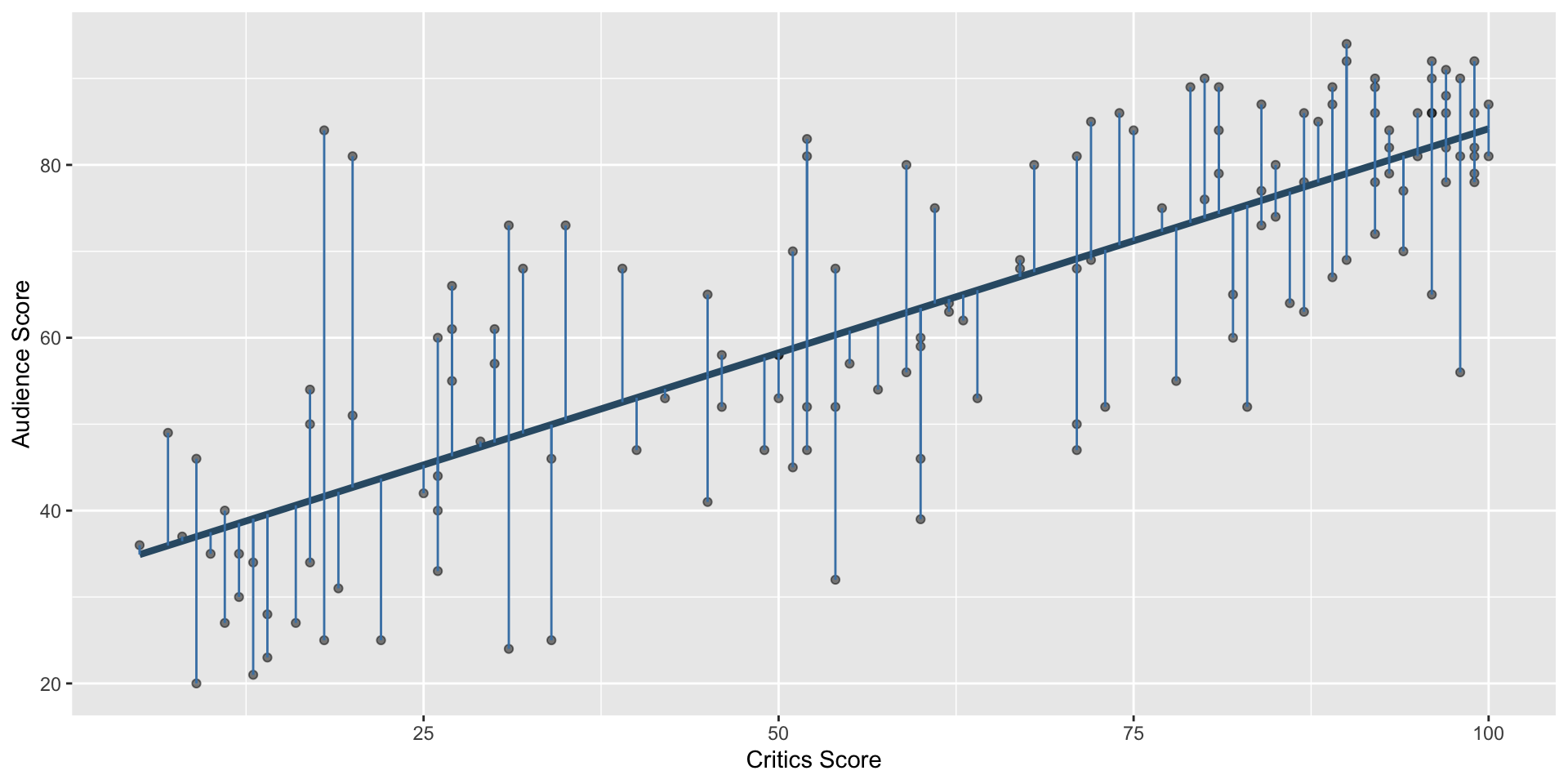
Least squares line

The residual for the \(i^{th}\) observation is

\[e_i = \text{observed} - \text{predicted} = y_i - \hat{y}_i\]

The sum of squared residuals is

\[e^2_1 + e^2_2 + \dots + e^2_n\]

The least squares line is the one that minimizes the sum of squared residuals

Least squares line

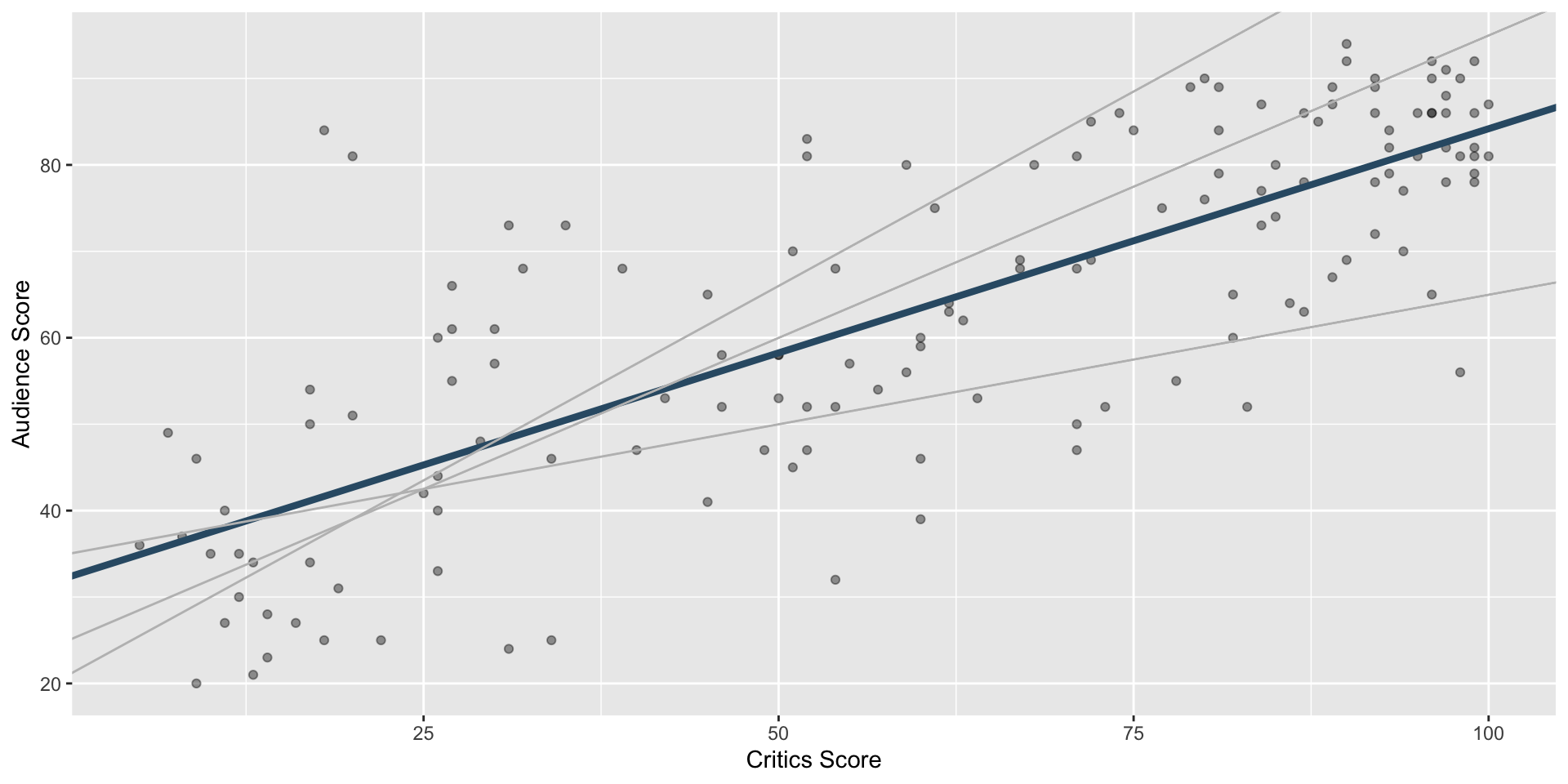
Slope and intercept

Properties of least squares regression

The regression line goes through the center of mass point (the coordinates corresponding to average \(X\) and average \(Y\)): \(b_0 = \bar{Y} - b_1~\bar{X}\)
Slope has the same sign as the correlation coefficient: \(b_1 = r \frac{s_Y}{s_X}\)
Sum of the residuals is zero: \(\sum_{i = 1}^n \epsilon_i = 0\)
Residuals and \(X\) values are uncorrelated

The slope of the model for predicting audience score from critics score is 0.519. Which of the following is the best interpretation of this value?

For every one point increase in the critics score, the audience score goes up by 0.519 points, on average.
For every one point increase in the critics score, we expect the audience score to be higher by 0.519 points, on average.
For every one point increase in the critics score, the audience score goes up by 0.519 points.
For every one point increase in the audience score, the critics score goes up by 0.519 points, on average.

Interpreting slope & intercept

\[\widehat{\text{audience}} = 32.3 + 0.519 \times \text{critics}\]

Slope: For every one point increase in the critics score, we expect the audience score to be higher by 0.519 points, on average.
Intercept: If the critics score is 0 points, we expect the audience score to be 32.3 points.

Is the intercept meaningful?

✅ The intercept is meaningful in context of the data if

the predictor can feasibly take values equal to or near zero or
the predictor has values near zero in the observed data

🛑 Otherwise, it might not be meaningful!

Application exercise

ae-13-modeling-penguins

Go to your ae project in RStudio.
If you haven’t yet done so, make sure all of your changes up to this point are committed and pushed, i.e., there’s nothing left in your Git pane.
If you haven’t yet done so, click Pull to get today’s application exercise file: ae-13-modeling-penguins.qmd.
Work through the application exercise in class, and render, commit, and push your edits.